Hello,
I have a question regarding the High Octane Strategy (https://www.portfolio123.com/port_summary.jsp?portid=1735193). Today is rebalance day and the holdings are:
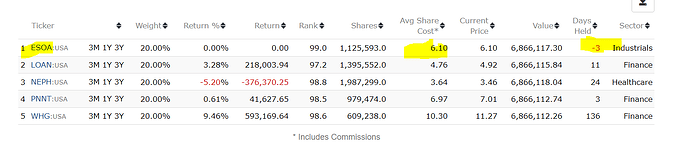
ESOA, LOAN, NEPH, PNNT and WHG. However, when I check the ranking with the exact universe that the buying constraints define, the top holdings are:
NEPH, SHAP, ARIZ, ALSA and CSLM. Can someone explain this discrepancy? Since Sell = 1 is set in the strategy, I would expect the top ranked stocks to be included in the portfolio after selling all others. The only interference in NEPH, all others are different.
Cheers,
Michael
Does the model basically cheat itself into a great performance?
It buys the stock at a price 3 days ago??! Really?
The strategy is ranking all the stocks in the North American universe and then eliminating all those that don’t pass the buy rules. If you create a DIFFERENT universe using the buy rules, the ranks are going to be completely different. To replicate the strategy by using the ranking, you’d have to run the rank first and THEN eliminate those stocks that don’t pass the buy rules.
This is a quirk of a Monday rebalance. All the “Days Held” are of Saturday but it’s actually buying the stock on Monday. It won’t show the proper numbers until Monday night after close because we don’t yet know what price it’ll pay for ESOA.
Hello Yuval,
thank you so much for your time and your answer. why is it that the ranks are then different. To me this doesn’t make sense: Why does it matter if I first take all stocks and eliminate all stocks with e.g. market cap < 500Mio $ or if I for eliminate all stocks with Mcap < 500 Mio AND THEN do the ranking?
It should not matter, I want to understand the math behind it…
Cheers,
Michael
Maybe the beginning of this article will help: How to Design a Fundamentals-Based Strategy That Really Works, Part Two: Applying Rules and Designing Ranking Systems - Portfolio123 Blog. The Nigeria/Ghana/Liberia analogy is most pertinent here.
After reading that, consider that many nodes are ranking by comparing companies to others in their industry and industries that are much much smaller in one universe than in another are going to get very different ranks. For example, if an industry has twenty stocks, the top two ranked stocks are going to get scores of 95 and 90; if it has only five stocks, the top ranked stocks are going to get scores of 80 and 60. The same logic applies to other nodes.
What Yuval said. It shows you the proposed trade. It puts in a price as a placeholder. And then backfills with the actual price when Monday’s trading is done.
It matters a lot. Use dividend yield as an example. Assume you only trade S&P 500 stocks.
If your universe is just S&P 500, the dividend yield factor will be more meaningful to distinguish between stocks with yields of 2-5%. Because most stocks have dividend yields in this universe. A rank of 75/100 is a 3.29% yield which is ticker SJM.
But now use the universe of all listed stocks. Because we are including OTC and microcaps, there are many stocks without a dividend yield. Therefore, any stock with a dividend yield will logically be ranked higher when compared to the parent universe. SJM now ranks as 85.48 / 100.
Now in a multi-factor ranking system, your dividend yield factor will have more influence if your universe is S&P 500. Any stock with a dividend yield will receive a decent rank if you use a very broad universe.
Thank you both for the answers. Makes a lot of sense and clarifies things. Highly appreciated!