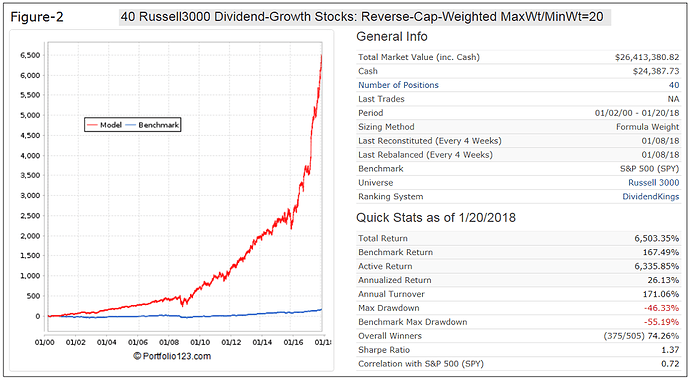
By now, many (or most or all) of you know I don’t advocate using any factor in isolation. An example I often use is Value, where I derive the ideal P/E as E / (R-G) where R is risk/quality and g is growth.
I did a quick back of the envelope empirical study to test some of this.
I started with the PRussell3000 universe.
I used a 5-years ago as of date (1/30/13)
I screened for stocks that back then Ranked above 90 for “Basic: Value”
So as of 1/30/13, all of these stocks could have been considered good value plays. And since the ranking system I used (it’s available as a pre-set on p123) is pretty generic, I figure any other ranking system would produce a list with very heavy overlap.
I then measured performance over the next 5 years; well, actually, since this is spit and chewing gum, I roughed it out by fast forwarding to today and computing close(0)/close(1200) assuming 240 trading days in a year.
After knocking out 16 stocks that didn’t have full 5-year histories, the average % return for the group was 63% and the median was 54%. But the maximum was +397% and the minimum was -91%. Remember, this range is only for stocks that were ranked above 90 for value. That’s a pretty big range and one I’m sure many have wrestled with as models were taken from backtest to live trading.
I then ordered the 90+ Value Rank 2013 stocks into five buckets based on the future 5-year returns that were actually delivered. For each bucket, I computed (as of today) the 5-year rates of sales and EPS growth tat actually occurred. Here are the results:
Bucket # Avg % Return Avg 5Y Rev Growth Rate Avg 5Y EPS Growth Rate
1 +171% +10.4% +20.0%
2 +98% +7.2% +15.4%
3 +56% +9.9% +8.3%
4 +19% +11.6% +3.1%
5 -32% +7.0% +3.0%
What does this mean for p123 users?
Obviously, this is not anything you can literally apply because I’m matching returns with KNOWN future growth rate (we see sales is meh but EPS growth is important). But it conforms the strategic roadmap I’ve been advocating. When it comes to value, don;t just look for good value. You also need clues that allow your models to make reasonable assumptions about future growth prospects. The difference between a successful and unsuccessful value strategy has little and probably nothing to do with the value rank you use. It probably depends more on how good a job you do (1) creating a pre-qualified list of companies against which you’ll apply your value ranks and/or (2) how good a combo rank you develop; one that effectively combines value with growth.
How do you model for clues to future growth? That’s the challenge. If I knew the answer, I’d be rich enough to own the world. But I suggest ways to move models in the right direction is to focus on clues provided by fundamental capacity for growth (returns on capital, etc.) and sentiment measures that let you tap into investment community expectations such as estimate revision, projections and good solid technical analysis. What about historical growth rates? I’ve had so-soi success at this, but it’s fertile ground for testing (Are growth rates persistent? If not, what might you discover and measure that might allow you to decide some growth rates are likely to be more persistent than others?)